Rocket Mechanics from Kerbal Space Program - Part 3
- mollievonehr
- Apr 4
- 10 min read
by Michael Lingg
Transfer to and from moons
Introduction
In our previous posts we discussed how to reach orbit (Part 1), and how to maneuver in orbit (Part 2). Now the concepts that were discussed previously will be used in traveling to and from moons. Travelling from a celestial body to its moon is an important activity in itself, furthermore what is learned here is foundational for more complex missions, such as interplanetary travel.
Understanding Moon Transfers
Moons are natural satellites orbiting a larger celestial body. To reach a moon from orbit around the larger body, we need to adjust our rocket’s orbit to intersect with the moon’s orbit when the moon will be near this intersection point. This process is relatively simple in KSP when travelling to the Mun, but can be more challenging when dealing with complex inclination changes and real life multiple body gravitational influences. Returning from the moon presents additional challenges, but is still quite manageable when the challenges are understood.

Transferring to a Moon

The image above shows a rocket in orbit around Kerbin, with the Mun orbiting at a higher altitude. To reach the Mun, we need to intercept its orbit and time our maneuver so that the Mun is at the interception point when our rocket arrives. In KSP this is straightforward, when the Mun is approximately 45 degrees ahead of the rocket in orbit (as shown in the image below), a prograde burn is performed until the rocket’s apoapsis reaches the orbit of the Mun.

The Mun's lack of inclination relative to Kerbin’s equator simplifies transfers. Since the KSC (Kerbal Space Center) launch pad sits right on Kerbin’s equator, a rocket launched due east will have an inclination that matches with the Mun. However, other moons, such as Minmus or Earth’s Moon, introduce complications by having an inclined orbit. Minmus has a consistent inclination of 6 degrees, while the Earth’s Moon can vary between 18 and 28 degrees.
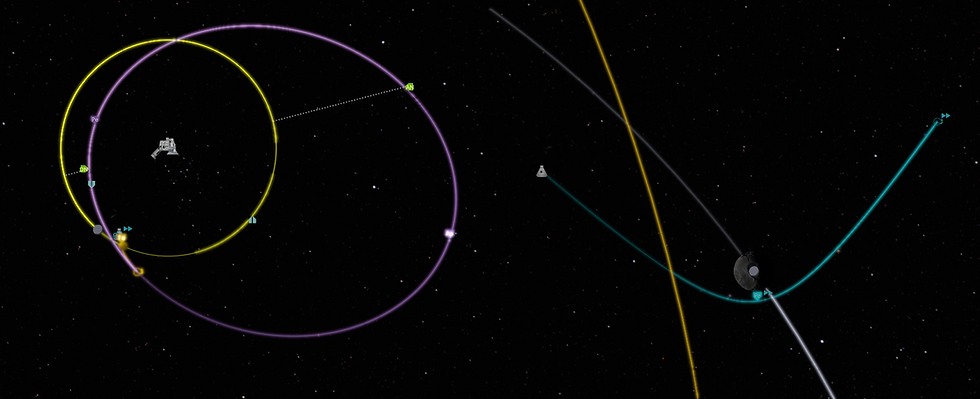
By carefully timing the launch, a rocket can lift off directly into an orbit with an inclination matching that of the moon. This requires lifting off when the launch point intersects the target body’s orbit (or is close to). In the image below, the purple line represents Minmus with its 6 degree inclination, passing through Kerbin’s equator with the barely visible KSC launch pad in the center of Kerbin’s image.

Launching when the launch point intersects Minmus’ orbit is possible in KSP as the Kerbal Space Center (KSC), where the launch pad is located, is at the equator, so inclined moons will intersect the launch pad at some point. The Kennedy Space Center (the KSP KSC is named to be reminiscent of Kennedy, I will not use Kennedy as an acronym) is located at 28.6 degrees N latitude, so the Moon can almost pass overhead, but never quite does. As a result, Apollo missions used a slightly different method that I will discuss later in the real world considerations.
Another option, if well timed, is to perform the Trans Lunar Injection (TLI) burn and inclination matching burn at the same time, when the TLI burn point is the same point as the point where the rocket and target body orbital planes intersect. Unfortunately windows for such a burn tend to be few and far between.
Personally, I rarely have the patience to get a perfectly timed inclination matching burn, as I am just flying a simulated rocket, so once in orbit I perform a burn to match my rocket’s inclination with the target when the rocket and target body orbital planes intersect. With my rocket in the correct inclination, I then burn prograde to intersect the moon as soon as the rocket reaches the right point in the orbit.
Now our rocket is on its way to intercept the Mun, if we were accurate enough, we are on the way to splatter our rocket all across the surface of the Mun.

Entering Moon Orbit
We likely want to avoid this crash, as we would instead prefer to enter orbit around our moon where we can make scientific observations. The most basic process for this is to complete a Hohmann, or bi-impulse, transfer to match the orbit of the Mun around Kerbin. However, KSP offers a simpler method of accomplishing this.
In the real world, all celestial bodies gravitationally affect a rocket in some manner. KSP simplifies this so the game can run on slower PCs, without looking like a powerpoint slideshow, by only simulating the gravity of the body with the greatest gravitational effect.
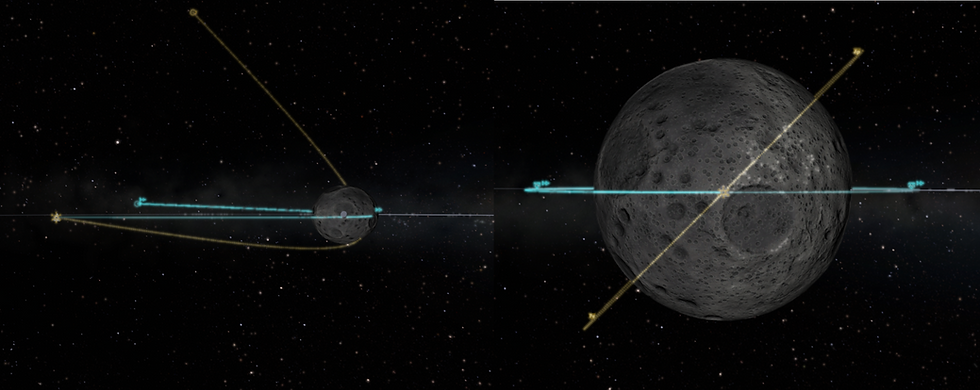
In the real world the sphere of influence (SOI) of a gravitational body is the sphere inside which the body’s gravitational influence significantly outweighs all other bodies, but other bodies will still have a gravitational effect. In KSP, an SOI roughly corresponds with reality, but the effects are different. In KSP, inside the Mun’s SOI the rocket will be gravitationally affected by the Mun but not by Kerbin or any other body. Outside the Mun’s SOI but inside Kerbal’s SOI, the rocket will be gravitationally affected by Kerbin, but not by any other body, including the Mun. The image below shows a rocket inside of Kerbin’s SOI (left, the rocket orbit is in purple), transitioning to the Mun’s SOI (right, the rocket Mun orbit is in blue, the orbit the rocket would follow after it exits the Mun’s SOI on the present trajectory is shown in yellow on the right side).
So in KSP we do not have to think about how we will complete the Hohmann transfer to circularize our orbit over Kerbin, in sync with the Mun. Instead, once we enter the Mun’s SOI we just have to use normal orbital maneuvering to adjust the rocket’s current trajectory (almost certainly a hyperbolic trajectory) to the desired orbit.
The first thing we likely want to do is to avoid the previously mentioned splattering of our rocket across the Munar(or lunar) landscape. There are two primary methods of accomplishing this. The first maneuver is accelerating prograde, which will increase the periapsis, but not very efficiently. The second method is burning Radial Out. As we discussed previously, Radial Out rotates the orbit around the current position of the rocket, away from the center of gravity. This method will efficiently raise the periapsis away from the moon, and should be continued until the periapsis is at the final desired orbit (Unless, unlike me, you performed your TLI burn accurately enough to require no correction once you enter the moon’s SOI. I’m not that good). The image below shows a Prograde burn for a rocket far out in a hyperbolic orbit on the left, while on the right is a Radial Out burn. The Prograde burn requires 200 m/s delta-v to reach a periapsis of 90 KM, while the Radial Out burn only uses a little over 25 m/s to achieve a periapsis of 100 KM.

In case you want a polar orbit, normal or anti normal maneuvers may be desired to change the rocket’s path to pass over a pole, and is the most efficient as far from the moon as possible. The image below shows how the inclination can be changed from far out in a hyperbolic orbit on the left on the left, while the right side of the image shows how the inclination can be changed in a circular orbit. The earlier inclination change is less costly, but also less precise for targeting a particular landing spot, particularly when the rotation of the moon has to be accounted for (NASA could compute the proper orbit from far out, my math isn’t that good).
The next step will typically be transferring into a stable orbit around the moon. This maneuver is trivial, burn retrograde at the periapsis (which now should be above the surface of the moon), until the orbit is circularized, or otherwise matches the desired final orbit. Lastly we can also adjust the inclination if we want to do this now to dial in a landing location.
Returning Home
Now that we’ve gone out and explored a moon, we would like to come home, if there are fleshy beings on board the rocket anyway. As of now, the Earth (and likewise in KSP, Kerbin) is the only place with breathable air, and we can only take a limited supply of air with us. More concerning to Kerbals (who in the default game have no life support needs), they might run out of snacks and have to wait for a rescue. Snacks are very important to Kerbals.
First we have to burn prograde to escape the moon’s gravity, or in KSP get out of the Mun’s SOI. This also depends on which direction the rocket is orbiting, we will use a retrograde orbit, as described below in real world considerations of the Apollo flight plans. The position within the orbit where we burn has an effect on how efficient (or possible) our return is. The image below shows the result of burning prograde when the rocket is:
On the farside of the Mun (upper left)
Heading toward Kerbin (upper right)
On the nearside of the Mun (lower left)
Heading away from kerbin (lower right)
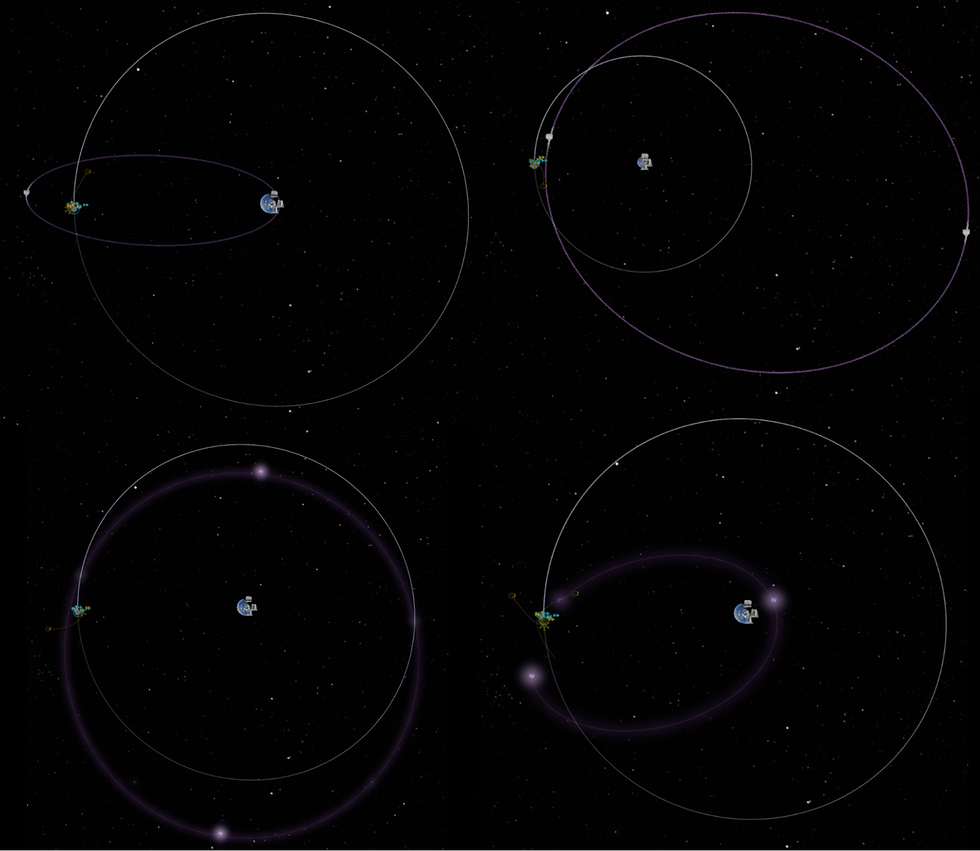
As you can see, when the rocket is on the nearside of the Mun and heading towards Kerbin, burning prograde to leave the Mun’s SOI will result in an orbit that travels further than the Mun’s orbit. When the rocket is angled away from Kerbin, or on the farside of the Mun, the lowest point of the orbit after leaving the Mun’s SOI will be closer to Kerbin. While burning prograde while the rocket is on the farside of the Mun can result in reentering Kerbin at the desired altitude, it is not the most efficient as this burn exits the Mun’s SOI with an angle slightly perpendicular to the Mun’s orbit around Kerbin. Burning prograde about half way between when the rocket is pointing away from Kerbin and when the rocket is on the farside of the Mun is the most efficient as the rocket will exit the Mun’s SOI on a parallel course retrograde (opposite) to the Mun’s orbit around Kerbin.
Interestingly, most of what I find on the return burn from Earth’s Moon, known as Trans Earth Injection (TEI) burn, simply states it is performed on the far side of the Moon. I suspect that the return from the Moon has the same profile as the return from KSP’s Mun, and the actual burn on the farside of the Moon is actually about forty five degrees before the actual opposite point in orbit from Earth, which is still a point where the Moon blocks communications with the Earth. Of course KSP is a game, so it is possible some factors exist such that the TEI burn is performed in lunar orbit, fully opposite to the Earth.
Once we leave the Mun’s SOI, if we have a reasonable periapsis returning to Kerbin, all that is needed is to fine tune the periapsis for reentry. As the landing point is not overly important in KSP, we simply need to burn prograde or retrograde (a slight radial burn can be most efficient, but I don’t optimize this far) to achieve our desired periapsis. This is important as if the reentry is too high, the rocket will not slow enough from atmospheric friction to avoid flying back out into space. This is illustrated in the image below where the reentry pod is in the atmosphere after returning from the Mun, the altitude is nearly out of the atmosphere (Kerbin’s atmosphere ends at 70 KM) and the velocity dial at the top shows the reentry vehicle climbing at a high rate of speed.

Too high of a reentry does not mean the rocket will fly off into space forever, the rocket will complete an orbit and return to the atmosphere at the next periapsis. This will likely require multiple orbits, and dips into the atmosphere, before it slows enough to be captured by the atmosphere. If you have plenty of time, and supplies, this can be fine, but likely this will take too long for the safety of the Kerbalnauts (or astronauts).
On the other hand, if the return periapsis is too low, the rocket will still have an extremely high velocity as it passes through dense air, causing rapid friction heating and the rocket will burn up, as shown in the image below.

This necessary balance for reentry leads to the optimal altitude having enough air density to slow the rocket enough that it will not return to space, yet also not burn up during reentry. If you have successfully performed all of these stages, your Kerbalnaut is happily sitting back on Kerbin after their trip from a moon.
Real World Considerations
Computing the Transfer Window
In the real world, we would rather not just say burn prograde when the moon is over thattaway. So there are specific computations to determine when to begin the transfer to the moon.
The first step of the equation is to compute or the semi-major axis of the orbit:

where:

The second step is to use the value from the first step to compute the transfer time for the rocket using Kepler’s third law:
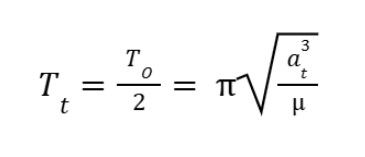
where:

Finally this is used to compute the phase angle, or how far behind in the orbit the rocket needs to be to catch up with the moon while transferring to the higher orbit:

where

Apollo Launches
Apollo missions to the Moon were a lot more complicated than a KSP flight to the Mun. As mentioned before the Moon’s orbit does not quite take it over the Kennedy Space Center. So Apollo would launch when the Kennedy Space Center was as close as possible to the Moon’s orbital plane, but this would never be perfect. This would typically result in a single launch window each day. The launch would not be due east, but at an angle to match the Moon’s inclination as best as possible.
After launch, Apollo would enter a parking orbit around the Earth to assess the spacecraft and determine if they were ready to transfer to the Moon. Once the spacecraft was ready, a mostly prograde TLI burn would be performed to raise the spacecraft apoapsis to intersect with the Moon, using a computation similar to the one above. Mid course corrections would then be used to make any remaining corrections to inclination, or other errors in the flight path.
Free Return Trajectory
Another method NASA used in some missions flying to the Moon was the free return trajectory. In this maneuver the spacecraft would initially be aimed to pass behind the Moon, using the Moon’s gravity to curve the spacecraft’s flightpath to a proper approach to return to Earth. This approach likely would not be perfect, but the required corrections would be minimal.
If the decision was made to orbit the Moon, a correction burn would be made to aim the spacecraft to fly across just in front of the Moon, leading to a gravity assist to slow the spacecraft relative to the Moon, possibly resulting in a highly elliptical orbit around the Moon. From here, less fuel is needed to enter the desired final orbit.
Curiously, most figures for the free return trajectory show the figure eight pattern used when the spacecraft will actually enter orbit around the Moon. I believe the following figure is an accurate depiction of the free return trajectory, along with the transfer maneuver that would be used to switch from a free return trajectory, to a path to enter orbit.
Direct Ascent
Another approach to a moon that was considered, but ultimately rejected by NASA, is the direct ascent approach. Here the rocket lifts off, and rather than entering orbit, continues to accelerate on a trajectory to intercept the moon. I believe this approach would require less fuel (depending on staging) as it could take better advantage of the Oberth effect and have less gravity loss. This approach was rejected by NASA as the method would require too much thrust early on during the launch, as well as being rather inflexible as to the flight plan. NASA chose a flight plan with a parking orbit that provided improved safety and flexibility.






Comments